WHAT DO BUTTONS, DARTBOARDS, A BLACK FOREST CAKE AND THE COLOSSEUM HAVE IN COMMON?
Exactly, they are round. More precisely, circular. Or even better: If you measure their circumference and divide it by their diameter, you always get one number: π, pi.
Pi (π) is one of those famous constant numbers that keep popping up in all sorts of different fields. Many calculations, many formulas, and most things that feature a circle ultimately depend on its 3.141592…. Even those who are searching for Nessie bump into.
FINDING NESSIE
PI (Π) IN CITIES AND PEOPLE
Let’s leave Nessie alone again, nevertheless appears almost everywhere. Besides circles and countless mathematical formulas, you can find it playing pool. Even in the Bible. As well as in cities. And it works like this:
If you randomly distribute points in a circle with radius r, you you may be (more or less) stunned (depending on whether you are stunned by at all) to find the following: The average distance between points is 128r/45. The same formula is used over and over to model various aspects of urban transportation and traffic flow. For more than 40 years, we have been modeling cities using the same formula.
COMMUTING BY PI
If you know the area of a city, say A, then traveling from one location to another will grow according to 128√A/45π. This formula holds for cities that are perfectly circular (perhaps a very simple city!) and where you can travel in a straight line between any two points. Of course, no city is like this, but it gives us an idea of how long trips will be and how long the commute will take.
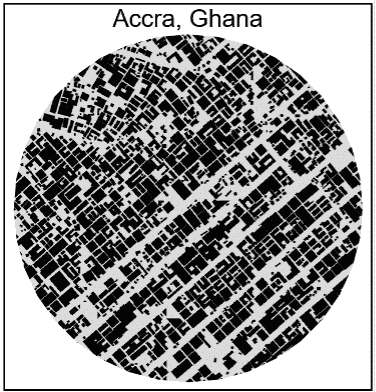
Vienna, for example, has an area of roughly 415 km2. So if we take any two locations in the city, they are on average 18 km apart. However, Innsbruck has a surface of 105km2, so on average the places are 9 km apart. This is shown in a recent study by Rafael Prieto-Curiel of the Complexity Science Hub, who, together with colleagues, measured about 183 million buildings in African cities. He used a similar formula to the one for the distance between points inside a circle can also be used to measure distances between buildings in a city.
There are other aspects of cities, including their irregular shapes, the space between its buildings, the many roads or its fractal patterns. However, keeps appearing!
Last but not least, does not even stop at the city boundaries. Instead, the fascination with lives in the heads of people. This culminates in worldwide competitions in which people try to outdo each other in enumerating more digits of . The official world record is currently 70,030 digits. It took Suresh Kumar Sharma 17 hours and 14 minutes.
This was in 2015, and no one has officially beaten it since. But developer Emma Haruka Iwao announced another record on June 8, 2022: a program from Google calculated the circular number to 100 trillion digits. That took 157 days. By way of comparison, in 1940 scientists were only able to calculate around 1,000 decimal places.
Happy day! A day for mathematicians, people who like math, or even people who suffered from in school but can still remember the first digits of 3.14!
Imagine a person trying to take the best photo of Nessie, the Loch Ness monster. Hundreds of people have tried it. The lake itself is quite large (a surface of 56 km2). So, not an easy task. Where would you start looking for Nessie? The best technique: where it was last seen. At least you know that Nessie once stayed in that part of the lake.
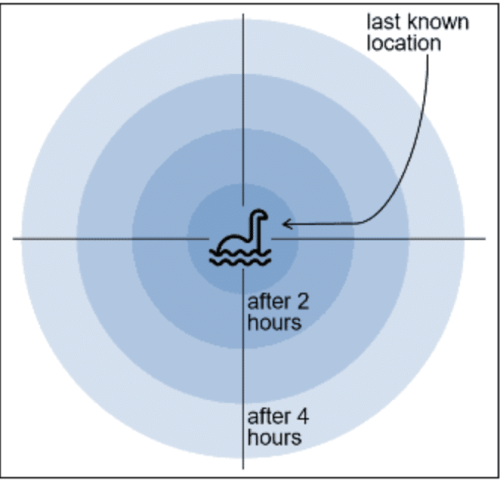
The issue is that the monster moves, and even if it moves slowly, the area that we have to search grows pretty quickly. For example, if Nessie moves at a speed of 1 km per hour, what is the area we need to search? Just one hour after the last known report, the monster could be anywhere within a circle with an area of 3.14 km2. And in two hours, the area will be 12.6 km2. So the area where the monster is sure to be after h hours since its last sighting is πh2.